Light fields are image-based representations that use densely sampled rays as a scene description. In this paper, we explore geometric structures of 3D lines in ray space for improving light field triangulation and stereo matching. The triangulation problem aims to fill in the ray space with continuous and non-overlapping simplices anchored at sampled points (rays). Such a triangulation provides a piecewise-linear interpolant useful for light field super-resolution. We show that the light field space is largely bilinear due to 3D line segments in the scene, and direct triangulation of these bilinear subspaces leads to large errors. We instead present a simple but effective algorithm to first map bilinear subspaces to line constraints and then apply Constrained Delaunay Triangulation (CDT). Based on our analysis, we further develop a novel line-assisted graph-cut (LAGC) algorithm that effectively encodes 3D line constraints into light field stereo matching. Experiments on synthetic and real data show that both our triangulation and LAGC algorithms outperform state-of-the-art solutions in accuracy and visual quality.

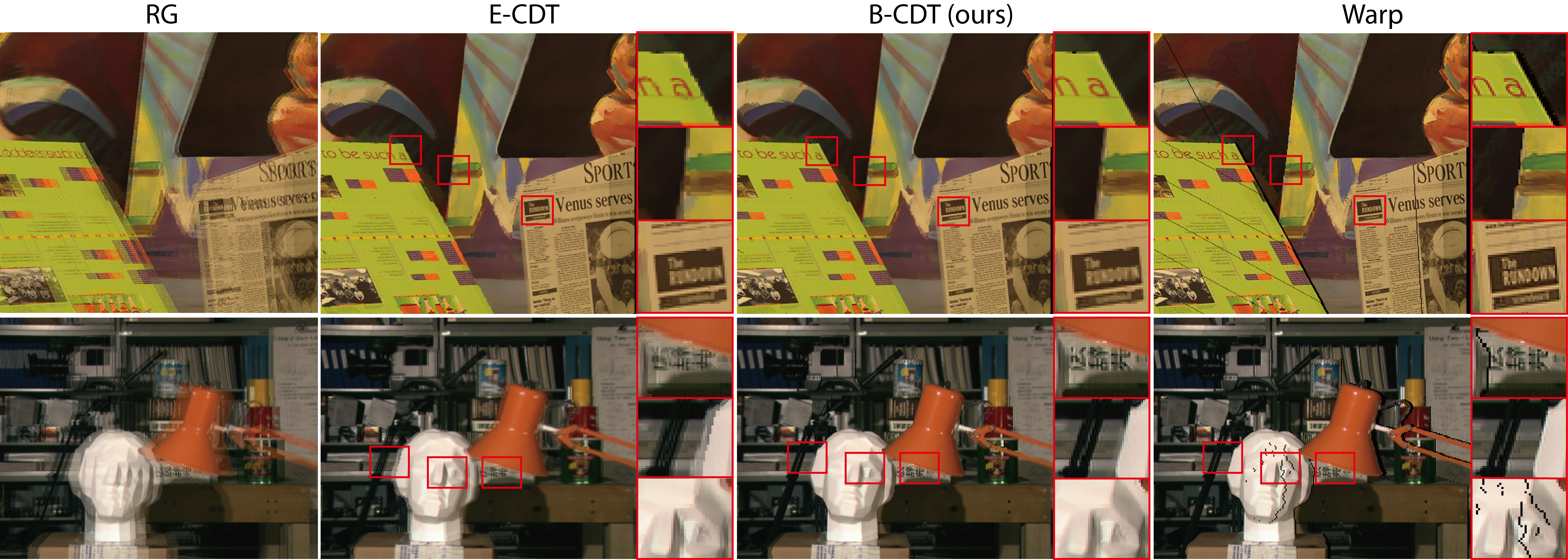
E-CDT: EPI Constrained Delaunay Triangulation
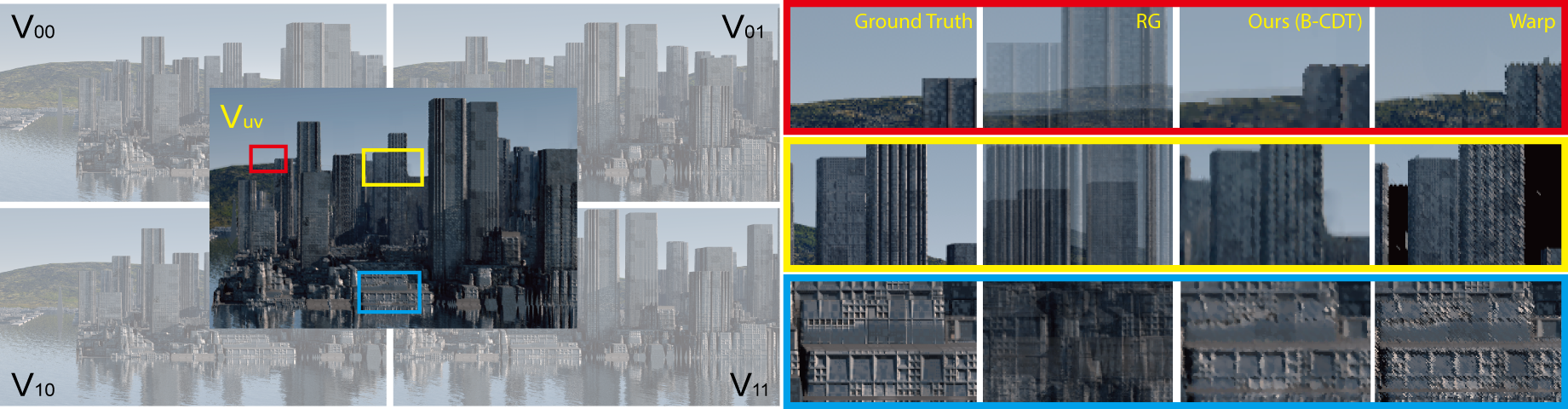
B-CDT: Bilinear Constrained Delaunay Triangulation

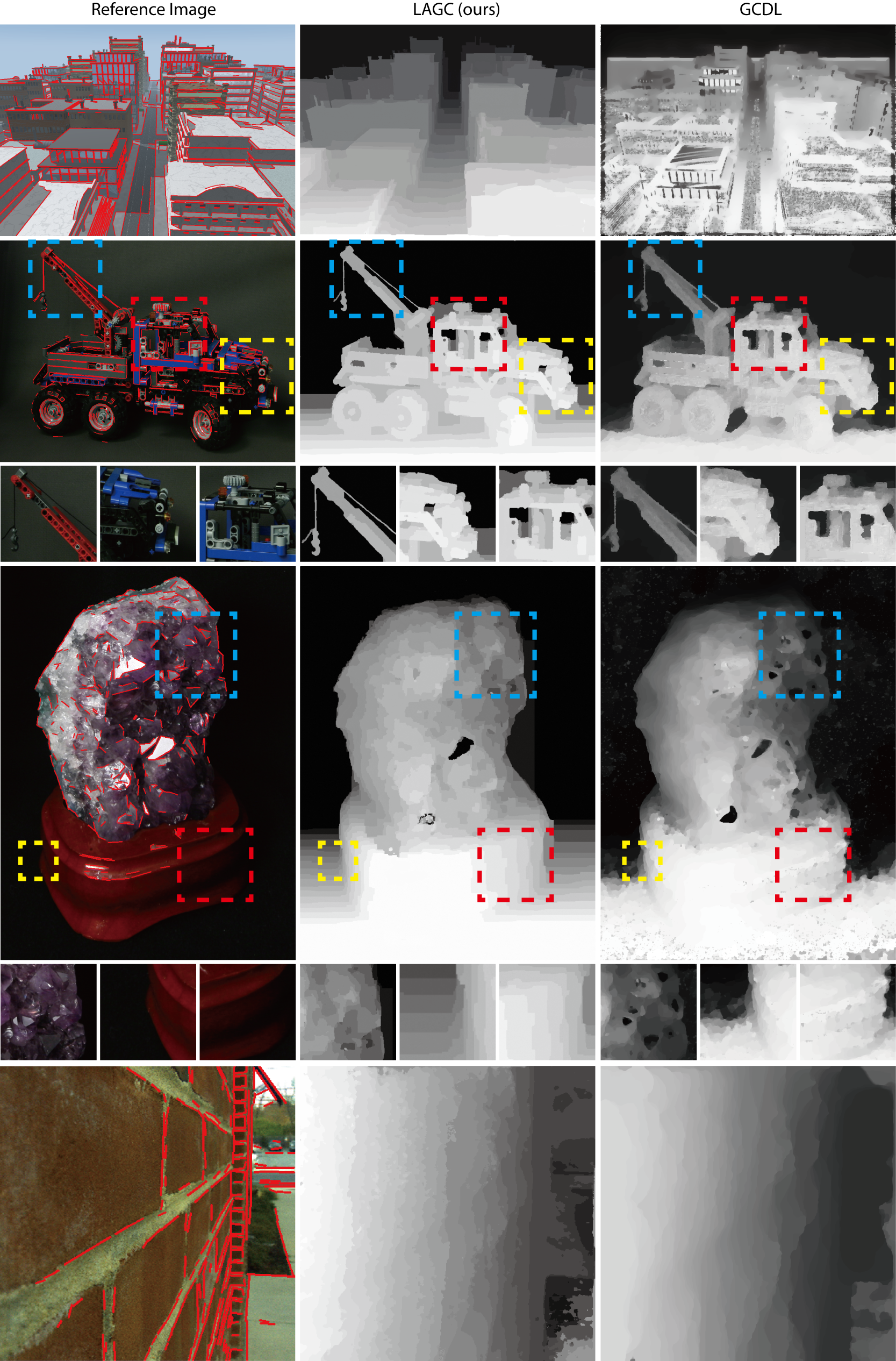
GCDL: Global Consistent Depth Labeling [Wanner and Goldlücke '12]